Using lengths to represent equality
Materials
Cuisenaire rods or paper strips cut to whole centimeter lengths
Actions
Students work in pairs.
One student puts a few rods (or strips) end-to-end.
The other student matches that length with a different combination of rods (or strips).
When two different ways of making the same length are found, the students write a number sentence reflecting the equality.
For example, if the first student uses a rod of length 4, a rod of length 2 and a rod of length 1 and the second student uses three rods of length 2 and a rod of length 1 (as shown below), they should write 4+2+1=2+2+2+1.
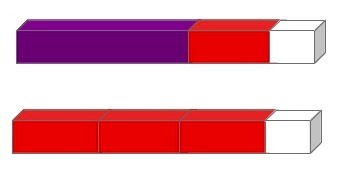
{\Large 4+2+1=2+2+2+1}



