Largest Number Game
Dona had cards with the numbers 0 to 9 written on them. She flipped over three of them. Her teacher said:
If those three numbers are the digits in another number, what is the largest three-digit number you can make?
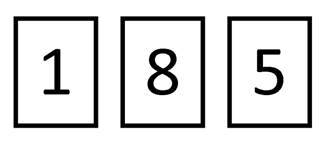
- First Dona put the 8 in the hundreds place. Is this the right choice for the hundreds place? Explain why or why not.
- Next, Dona said, “It doesn’t matter what number I choose for the other places, because I put the biggest number in the hundreds place, and hundreds are bigger than tens and ones.” Is she correct? Explain.
