Kiri's Multiplication Matching Game
The game is played with one to four players.
To play, two decks of cards are needed: the "matching" cards and the "target" cards.
An array of matching cards, which have numbers on their faces, is dealt face down. There can be twelve to twenty-four cards in the array. A single target card is dealt face up.
A turn consists of a student flipping over matching cards one at a time, then trying to combine them to meet the criterion of the target card. The quantity of flipped cards and method of combination can be varied to utilize different skills and give variety to the game. Two possible versions of the game are described below. The student wins a point if they state a correct mathematical relationship between the matching cards and the target card.
-
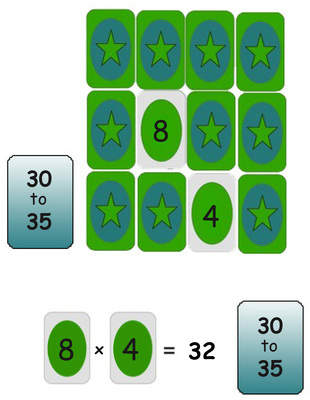
In one version, a player reveals two cards each turn. The player wins a point if they can produce a product which matches the target card. Those who wish to keep track of points can do so by keeping target cards that are correctly "matched". Target cards can be single numbers but will more generally be descriptions of possible products. For example, the target card could be $24$, in which case the player would be looking for pairs $6$ and $4$, $8$ and $3$, or $12$ and $2$. But the target could also say "$30$ to $35$". In this case, if a player turns over a $4$, for example, they would then need to try to find an $8$, and to win the point they would need to say "$8$ times $4$ is $32$, which is between $30$ and $35$."
-
Another version of the game lets each player reveal three cards each turn. The player wins a point if they can produce an equation with their three cards and the target card that involves any of the operations of addition, subtraction, multiplication, or division. For example, suppose the target card is $7$ and the player turns $15$, $3$, and $2$. The player can win a point by stating that $15 \div 3 + 2 = 7$.
In each case, once a target card is "matched", a new target card is revealed. If a target card is not matched, the next player has a chance to match it.
Both of these versions of Kiri's Mathematical Match Game could be played as no-memory, light-memory, or memory games.
- In the no-memory version, the chosen matching cards are left face-up after they are revealed.
- In the light-memory version, the chosen matching cards are left face-up until some player is able to "match" the target card and wins a point. When a match is made, all of the matching cards that are face-up are turned back face-down, the board is replenished, and a new target card is drawn, so the next player sees the initial set-up.
- In the memory version, the chosen matching cards are turned back face-down after every turn, so students would need to remember the locations of previously overturned cards in order to make use of them.
