Task
Part 1:
There are two design proposals for a new rectangular park in town.
- In design one, $\frac{3}{4}$ of the area of the park is going to be a rectangular grass area and $\frac{1}{2}$ of the grass area will be a rectangular soccer field.
- In design two, only $\frac{1}{2}$ of the park is going to be a rectangular grass area and $\frac{3}{4}$ of the grass area will be a rectangular soccer field.
Which design (one or two) will have a bigger soccer field? Explain your answer. Draw a diagram that can be used to compare the size of the soccer field in the two designs. Label the values $\frac{1}{2}$ and $\frac{3}{4}$ on the diagram.
Part 2:
Presley and Julia are cutting 1 ft. square poster board to make a sign for the new park. Presley cut her poster so that the length of the top and bottom are $\frac{1}{2}$ ft and the length of the sides are $\frac{3}{4}$ ft. Julia cut her poster so that the lengths of the top and bottom are $\frac{3}{4}$ ft and the length of the sides are $\frac{1}{2}$ ft.
Draw a diagram of each poster board. Label the values on the diagram.
How are their poster boards similar and different? Justify your reasoning.
IM Commentary
Part 1 of this task is designed to elicit student thinking about multiplication of fractions and the commutative property. An entry-level task into the concept, students can solve the problem without recognizing that they can multiply the fractions to find the fraction of the park that is the soccer field. However, they should have seen prior to this task that $\frac{3}{8}$ can be interpreted as one-half of three-fourths of a whole, so that they can make the connection between the two designs and the expressions $\frac{3}{4}\times\frac{1}{2}$ and $\frac{1}{2}\times\frac{3}{4}$. Through discussion of the task and student solutions, students can extend and apply their previous understandings of multiplication of whole numbers and their growing understanding of the meaning of fraction multiplication to see that the commutative property still holds when multiplying fractions.
After students have solved Part 1, the class could have a discussion of the solution methods. Once both designs have been analyzed, the teacher could ask what the students notice about the solutions (they are the same). Either the students or the teacher should represent this discovery symbolically:
$\frac12 \times \frac34 = \frac34 \times \frac12$
The fractions $\frac{3}{4}$ and $\frac{1}{2}$ were selected so that students should have an understanding of both values, providing students with multiple entry points and opportunities to reason and make sense of the problem. Considering how the fractions might be partitioned and represented was also taken into account. Substituting other fractions within the problem may elicit different opportunities for learning.
Part 2 of the task uses the area of a rectangle to help students understand why the commutative property always holds. In part 1, students see that $\frac{1}{2}$ of $\frac{3}{4}$ gives the same results as $\frac{3}{4}$ of $\frac{1}{2}$, and Part 2 asks students to focus on a rectangle with side-lengths $\frac{1}{2}$ and $\frac{3}{4}$. A teacher led-discussion that helps students see the relationship between the diagrams from Part 1 and Part 2, in particular, that you can see the answer in part 1 corresponds to a rectangle like those drawn in part 2. Note that the difference in the contexts and the diagrams for parts 1 and 2 are subtly different, but seeing this difference is very important for students’ future mathematical lives. If we analyze the two parts using units, we might write
$\frac{1}{2}\times\frac{3}{4}\mathrm{square\ units}=\frac{3}{8}\mathrm{square\ units}$
For the first one and
$\frac{1}{2}\mathrm{ft}\times\frac{3}{4}\mathrm{ft}=\frac{3}{8}\mathrm{square ft}$
for part two.
The first of these corresponds to thinking of multiplication as scaling and the second corresponds to multiplication and area. Students will build on these foundations in later grades when they study the relationship between multiplication, ratios, and rates in middle school and unit analysis in high school.
This task was written as a part of a collaborative project between Illustrative Mathematics, the Smarter Balanced Digital Library, and the Teaching Channel.
Solution
Part 1:
The two designs have the same size area. Students may use a variety of representations to model the soccer fields, one of which might include a diagram like the one below.
Design 1:
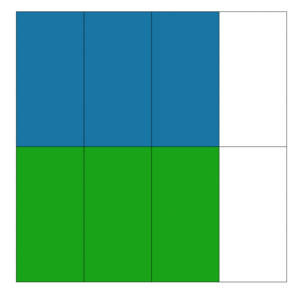
Color represents the grass and blue represents the soccer field. $\frac{1}{2}$ of $\frac{3}{4}$ is $\frac{3}{8}$.
Design 2:
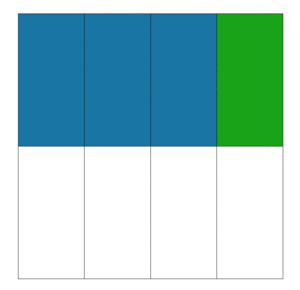
Color represents the grass and blue represents the soccer field. $\frac{3}{4}$ of $\frac{1}{2}$ is $\frac{3}{8}$.
When discussing this part of the task, these diagrams should be the ones that are the focus of a whole-class discussion, as they tie into the diagrams for Part 2 in fundamental ways.
Students may use equations to represent and solve the problem. Such equations would include $\frac{3}{4}\times\frac{1}{2}=\frac{3}{8}$ or $\frac{1}{2}\times\frac{3}{4}=\frac{3}{8}$. They may identify the commutative property as a way to justify the soccer fields being the same size.
Part 2:

Note that the only difference between the two rectangles is that one is a rotation of the other. In particular, they have the exact same area. Note that the area also corresponds to the same areas that we saw in Part 1 by finding $\frac{1}{2}$ of $\frac{3}{4}$ and $\frac{3}{4}$ of $\frac{1}{2}$.



