Task
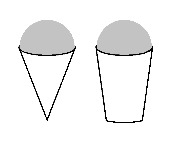
Pablo's Icy Treat Stand sells home-made frozen juice treats as well as snow-cones. Originally, Pablo used paper cone cups with a diameter of 3.5 inches and a height of 4 inches.

Conical Cup A
His supply store stopped carrying these paper cones, so he had to start using more standard paper cups. These are truncated cones (cones with the "pointy end" sliced off) with a top diameter of 3.5 inches, a bottom diameter of 2.5 inches, and a height of 4 inches.

Cup B
Because some customers said they missed the old cones, Pablo put a sign up saying "The new cups hold $50\%$ more!" His daughter Letitia wonders if her father's sign is correct. Help her find out.
How much juice can cup A hold? (While cups for juice are not usually filled to the top, we can assume frozen juice treats would be filled to the top of the cup.)
How much juice can cup B hold?
By what percentage is cup B larger in volume than cup A?
Snow cones have ice filling the cup as well as a hemisphere of ice sticking out of the top of each cup. How much ice is in a snow cone for each cup?
By what percentage is the snow cone in cup B larger than the snow cone in conical cup A?
Is Pablo's sign accurate?
IM Commentary
Students will just be learning about similarity in this grade, so they may not recognize that it is needed in this context. Teachers should be prepared to give support to students who are struggling with this part of the task. To simplify the task, the teacher can just tell the students that based on the slant of the truncated conical cup, the complete cone would be 14 in tall and the part that was sliced off was 10 inches tall. (See solution for an explanation.)
There is a worthwhile discussion to be had about parts (c) and (e). The percentage increase is smaller for the snow cones than it was for the juice treats. The snow cones have volume which is equal to those of the juice treats plus the volume of the dome, which is the same in both cases. Adding the same number to two numbers in a ratio will always make their ratio closer to one, which in this case means that the ratio - and thus percentage increase - would be smaller.
Algebraically, we can call the volume of the truncated conical cup $T$, the volume of the conical cup $C$ and the volume of the snow dome $D$. So from the computations in the solution we have, for example, that $\frac{T}{C}$ is roughly $2.23$.
But we only need to know that $T \ge C$ or $\frac{T}{C} \ge 1$, to deduce that $\frac{T + D}{C + D} \le \frac{T}{C}$.
To see this we compare
$$(T+ D) \times C = TC + DC$$ with $$T \times (C + D) = TC + TD,$$ and
see the latter is larger because $CD \le TD$, which we know because $C\le T$. Because the ratio has decreased when adding $D$ to the numerator and denominator, the percentage difference has also decreased.
Indeed, if the "dome" part were even larger, the percentage increase would be smaller. If the dome were large enough, Pablo's sign would need to be changed (in reference to the snow cones) because there would be less than $50\%$ more in the truncated cone cup.
Submitted by Michelle Mourtgos of Grantsville Junior High School to the fourth Illustrative Mathematics Task Writing Contest.





