The Lighthouse Problem
Task
Suppose that even in perfect visibility conditions, the lamp at the top of a lighthouse is visible from a boat on the sea at a distance of up to 32 km (if it is farther than that, then it is obscured by the horizon).
- If the "distance" in question is the straight-line distance from the lamp itself to the boat, what is the height above sea level of the lamp on top of the lighthouse?
- What are two other interpretations of the distance being investigated in this problem? Describe how to solve the alternate versions.
IM Commentary
In addition to the purely geometric and trigonometric aspects of the task, this problem asks students to model phenomena on the surface of the earth. This first step, observing that the bound of 32km on the visibility of the lighthouse is due to the curvature of the earth, will likely be a difficult part of the modeling process. Instructors should expect to provide guidance for this part, and possibly also the diagram in the solution.
The instructor can also choose whether or not to provide the radius of the earth, or to have students discover its relevance and then look it up in an available resource. Students may discover that since the earth is not a perfect sphere, the "radius" of the earth varies from one location to the next. As such, students may end up with one of many possible such values, with which the calculations in the solution could be repeated verbatim.
This problem is freely translated from the math and physics problem column in La Presse de Tunisie (October, 2006). It asked only the first question. La Presse is a general circulation newspaper in Tunis and this was the easiest of the three math problems. Presumably the first interpretation of ‘distance’ was intended in the original problem. But the variants give an opportunity for students to see the differences in the three values and use several trigonometric approaches.
It is tempting in b.ii to actually solve for $\theta$ and divide by $\cos{\theta}$. Not only is this more work but it might introduce round off error.
-
One should note that the small differences in the three answers are dwarfed by the approximations implicit in estimating the radius of the earth and the rounding off to even kilometers in stating the problem. In particular, the calculated differences between part a and b.i of less than 1/1000th of a meter and between b.i and b.ii of 1/100th of meter are negligible.
Solution
-
Consider the triangle with vertices $C$, the center of the earth, $A$, the lamp, and $B$, the boat. The radius of the earth can be found on the internet as 6,371 km.
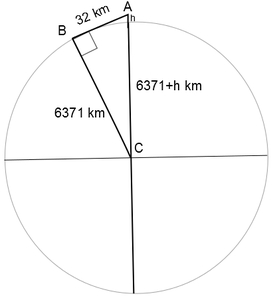 (Note that the diagram is drawn to a highly disproportionate scale to aid with visibility.)
(Note that the diagram is drawn to a highly disproportionate scale to aid with visibility.)
With notation as above, we have $AC = 6371+h$, $BC = 6371$ and $AB = 32$. Since we are told that the lighthouse can be seen from up to 32 km, $CBA$ is a right-angle. By the Pythagorean theorem, $$ 6371^2+32^2=(6371+h)^2=6371^2+2(6371)h+h^2, $$ which simplifies to $h^2 + 2(6371)h = 32^2,$ which in turn can be solved by the quadratic formula: $$ h=\frac{-12742\pm\sqrt{12742^2-4(1)(-(32^2)}}{2} = .080363643 \text{ km or } 80.36 \text{ meters}. $$ -
For the sake of measuring the discrepancy in our answer coming from different interpretations of "distance," we maintain a grossly unreasonable level of precision for this part of the problem.
Let $D$ be the base of the lighthouse, $R$ the radius of the earth and continue the notation of part a). Let $\theta$ be the angle between $ADC$ and $BC$. Then $\frac{R}{R+h}=\cos{\theta}$ so $h = \frac{R}{\cos{\theta}}-R$.
The other two interpretations are given below.-
Take the distance to be the length of the circular arc from $D$ to $B$. Then $\frac{\theta}{360}=\frac{32}{2\pi6371}$ or $\theta = \frac{360 \cdot 32}{2\pi6371}=.2877829139$ degrees.
Now the height of the tower is $\frac{6371}{\cos(.2877829139)}-6371=.0803649948 \text{ km}$, or approximately 80 meters.
-
Take the distance to be the straight-line distance from the boat to the base of the lighthouse, so $BD=32$. We calculate the angle $\theta$ using the law of cosines applied to the triangle $BCD$. We have $BD^2+DC^2=2(BC)(BD)\cos{\theta}$. So, $$ \cos{\theta}=\frac{BD^2-(BC^2+DC^2)}{-2(BC)(BD)} $$
or in our case
$$ \cos{\theta}=\frac{32^2-(2R^2)}{-2R^2}=.9999873859 $$The height of the lighthouse is $\frac{6371}{.9999873859}-6371=.0803654448 \text{ km}$ or 80.37 meters.
For comparison purposes, we continue to find $\theta$. Substituting, $\cos^{-1}(\theta)=.9999873859$ we see $\theta = .2877832164$ degrees which agrees to 4 places with the arcsin calculation.
-
The Lighthouse Problem
Suppose that even in perfect visibility conditions, the lamp at the top of a lighthouse is visible from a boat on the sea at a distance of up to 32 km (if it is farther than that, then it is obscured by the horizon).
- If the "distance" in question is the straight-line distance from the lamp itself to the boat, what is the height above sea level of the lamp on top of the lighthouse?
- What are two other interpretations of the distance being investigated in this problem? Describe how to solve the alternate versions.
