Are the Triangles Congruent?

Task

In each of the following diagrams, two triangles are shaded. Based on the information given about each diagram, decide whether there is enough information to prove that the two triangles are congruent.

1. In the figure below, $ABCD$ is a trapezoid with $\overline{AB} \parallel \overline{CD}$, and $M$ is the midpoint of side $\overline{AB}$.
   
   
   
   
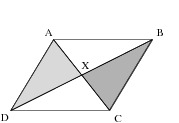
2. In the figure below, $ABCD$ is a parallelogram.
   
   
   
   
3. In the figure below, the circle is centered at point $O$, and the arc $AB$ is congruent to the arc $CD$.
   
   
   
   

IM Commentary

The purpose of this task is primarily assessment-oriented, asking students to demonstrate knowledge of how to determine the congruency of triangles. The solutions present two approaches whose appropriateness depends on the prior instruction for the class. The first is more suitable for classes which have spent time developing some of the fundamental theorems of geometry, using properties of parallelograms, central angles, congruency theorems, etc. The second is more appropriate for classes near the beginning their discussion of congruence, proves the congruence of the triangles by demonstrating a rigid motion that can be used to transform one of each pair of triangles to the other.

Solutions

Solution: Solution via Geometry Theorems

This approach to the solutions uses previously-discovered theorems about parallelograms. In particular, part (b) assumed students know facts about parallelograms (either that there opposite edges are congruent, or that their diagonals bisect each other), part (c) assumes knowledge about central angles, and both assume knowledge of the triangle congruence theorems.

1. Given the lack of information on $C$ and $D$, we cannot determine if if the two triangles are congruent. For example, if we were to move $D$ in the diagram to the position directly underneath $M$, then we would still have $\overline{AB}\parallel\overline{CD}$ and $M$ the midpoint of $\overline{AB}$, but $\triangle AMD$ is a right triangle whereas $\triangle MBC$ is not. The only piece of congruence between the two triangles that we can deduce is that $AM\cong MB$.
2. The two triangles are congruent by SAS: We have $AX\cong CX$ and $DX\cong BX$ since the diagonals of a parallelogram bisect each other, and $\angle AXD\cong \angle BXC$ since they are vertical angles. Alternatively, we could use argue via ASA: We have the opposite interior angles $\angle DAX\cong \angle BCX$ and $\angle ADX\cong \angle CBX$ and $\overline{AD}\cong \overline{BC}$ since opposite sides of a parallelogram are congruent.
3. The two angles are congruent by SAS: Since they are all radii of the same circle, we have side congruences $OA\cong OD$ and $OB\cong OC$, and we have $\angle BOC\cong \angle \angle AOC$ since they are central angles subtending congruent arcs.

Solution: Solution via Rigid Motions

This solution approaches the task via rigid motions.

1. Given the lack of information on $C$ and $D$, we cannot determine if if the two triangles are congruent. For example, if we were to move $D$ in the diagram to the position directly underneath $M$, then we would still have $\overline{AB}\parallel\overline{CD}$ and $M$ the midpoint of $\overline{AB}$, but $\triangle AMD$ is a right triangle whereas $\triangle MBC$ is not. The only piece of congruence between the two triangles that we can deduce is that $AM\cong MB$.
2. Yes, the two triangles are congruent. Triangle $\triangle BXC$ is the result of rotating triangle $\triangle DXA$ by 180 degrees around the center $X$.
3. Yes, the two triangles are congruent. Triangle $\triangle BOA$ is the result of reflecting triangle $\triangle COD$ across the perpendicular bisector of $\overline{AD}$.