Do two points always determine a linear function?
Task
- Suppose P_1 = (0,5) and P_2 = (3,−3). Sketch P_1 and P_2. For which real numbers m and b does the graph of a linear function described by the equation f(x) = mx+b contain P_1? Explain. Do any of these graphs also contain P_2? Explain.
- Suppose P_1 = (0,5) and P_2 = (0,7). Sketch P_1 and P_2. Are there real numbers m and b for which the graph of a linear function described by the equation f(x)=mx+b contains P_1 and P_2? Explain.
- Now suppose P_1= (c,d) and P_2 = (g,h) and c is not equal to g. Show that there is only one real number m and only one real number b for which the graph of f(x)=mx+b contains the points P_1 and P_2.
IM Commentary
In a geometric context, two distinct points P_1and P_2 always determine a unique line in the Cartesian plane (this is one of the basic postulates of Euclidean geometry). Only the non-vertical lines, however, can be described by the graph of a function f(x) = mx + b. This task focuses on producing an explicit function f(x) in the case where the line is not vertical.
This problem allows the student to think geometrically about lines and then relate this geometry to linear functions. Or the student can work algebraically with equations in order to find the explicit equation of the line through two points (when that line is not vertical).
Solutions
Solution: 1
-
The graph of the linear function described by f(x) = mx + b contains (0,5) when the y-intercept of the graph, given by b, is equal to 5. So the linear functions whose graph contains (0,5) are those of the form f(x) = mx + 5 where m is a real number.
In order for the graph of f to contain (3,-3) we need -3 = f(3) = 3m + 5. Solving for m gives m = -\frac{8}{3}. So there is only one linear function whose graph passes through (0,5) which also passes through (3,-3), namely the one described by f(x) = -\frac{8}{3}x + 5, depicted below.
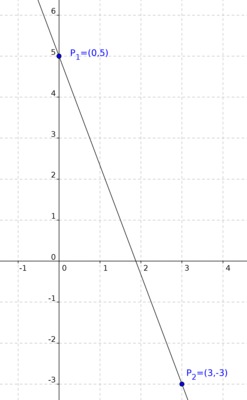
-
If f is any function, then its graph consists of the pairs (x,f(x)). So
for each x-value there can only be one associated f(x)-value which is
on the graph of f. In this case (0,5) and (0,7) both have x-value zero
and so these two points cannot be on the graph of any function, whether or not
the function is linear.

-
Asking for the point P_1 = (c,d) to be on the graph of f(x) = mx + b means that d = mc + b. Similarly asking for the point P_2 = (g,h) to be on the graph of f(x) = mx + b means that h = mg + b. Subtracting the second equation from the first gives d - h = m(c - g) so m = \left(\frac{d-h}{c - g}\right). Note that the expression for the slope m only makes sense if c \neq g, which has been assumed in the problem. Once we have found m we can plug this into one of the equations(either for d or for h) and then solve for b: d = \left(\frac{d - f}{c - g}\right) c + b. Solving for b gives b = d-\left(\frac{d-h}{c-g}\right)c. So with the hypothesis that c \neq g there is a linear function whose graph contains P_1 and P_2 namely f(x) = \left(\frac{d-h}{c-g}\right)x + d -\left(\frac{d-f}{c-g}\right)c.
Finally, we note that we have also that there is a unique such line, as any line containing P_1 and P_2 must have the slope and y-intercept as calculated above. Explicitly: If there were a different linear function g(x) = m_1x + b_1 whose graph contained P_1 and P_2, then by virtue of it containing P_1 we must have d = m_1c + b_1. Similarly, since the graph of g contains P_2, we must have h = m_1g + b_1. These two allow us to solve for m_1, as above, and we find the same solution, namely m_1 = \left(\frac{d-h}{c - g}\right). We then find, also as above, that b_1 = b and so g(x) = f(x): there is only one linear equation of the form f(x) = mx + b whose graph contains P_1 and P_2.
Solution: 2 Thinking Geometrically
From a geometric point of view, we can see the answer to part (c) as follows. Given the two points P_1 and P_2 we can draw the unique line containing these two points. If f_1(x) = m_1x + b_1 and f_2(x) = m_2x + b_2 were two equations whose graphs describe this line, this means that m_1x + b_1 = m_2x + b_2 for all values of x. This is only possible if m_1 = m_2 and b_1 = b_2 as we can see, for example, by rewriting this equation as (m_1-m_2)x = b_2 - b_1. The right hand side of this equation does not depend on x so this forces m_1 - m_2 = 0 and once m_1 = m_2 then the left hand side is zero, forcing b_1 = b_2.
Do two points always determine a linear function?
- Suppose P_1 = (0,5) and P_2 = (3,−3). Sketch P_1 and P_2. For which real numbers m and b does the graph of a linear function described by the equation f(x) = mx+b contain P_1? Explain. Do any of these graphs also contain P_2? Explain.
- Suppose P_1 = (0,5) and P_2 = (0,7). Sketch P_1 and P_2. Are there real numbers m and b for which the graph of a linear function described by the equation f(x)=mx+b contains P_1 and P_2? Explain.
- Now suppose P_1= (c,d) and P_2 = (g,h) and c is not equal to g. Show that there is only one real number m and only one real number b for which the graph of f(x)=mx+b contains the points P_1 and P_2.
