How many _______ are in. . . ?
Task
Solve each problem using pictures and using a number sentence involving division.
- How many fives are in 15?
- How many halves are in 3?
- How many sixths are in 4?
- How many two-thirds are in 2?
- How many three-fourths are in 2?
- How many \frac16’s are in \frac13?
- How many \frac16’s are in \frac23?
- How many \frac14’s are in \frac23?
- How many \frac{5}{12}’s are in \frac12?
IM Commentary
This instructional task requires that the students model each problem with some type of fractions manipulatives or drawings. This could be pattern blocks, student or teacher-made fraction strips, or commercially produced fraction pieces. At a minimum, students should draw pictures of each. The above problems are meant to be a progression which require more sophisticated understandings of the meaning of fractions as students progress through them. If the task is used to help students see the connections to the invert-and-multiply rule for fraction division (as described in the solution) then they should already be familiar with and comfortable solving Number of Groups Unknown (a.k.a. “How many groups?â€) division problems with visual models.
By helping students see how division is modeled through the use of visual models, students can develop a sense for how the fraction division algorithm is derived. The visual model for each problem can be connected to the algorithm for fraction division, and the carefully chosen sequence of problems shows the evolution of the algorithm (note that the types of pictures shown require different kinds of interpretations to see the connection to the algorithm). It also provides opportunities for the students to talk about the specific phenomena that fraction division results in a larger quotient when you divide by a number less than 1.
This task was submitted by Victoria Peacock to the fifth Illustrative Mathematics task writing contest.
Solution
-
Opening Question: How many 5’s are in 15?

There are three sets of 5 in 15.
Write this as a division problem: 15\div 5 = 3
You may want to do a couple of examples like this with whole numbers to familiarize students with thinking about division as how many of a number are in another number.
-
How many halves are in 3?

There are 6 half-sized pieces in 3 wholes.
Write this as a division problem: 3 \div \frac12 = 6
We can connect this to the algorithm for dividing fractions: 3 \div \frac12 = 3 \times \frac21 = 3\times2 and we can see that there are 3 wholes with 2 halves in each whole, so there are 3\times 2 = 6 halves in 3.
-
How many sixths are in 4?

There are 24 sixth-sized pieces in 4.
Write this as a division problem: 4 \div \frac16 = 24
We can connect this to the algorithm for dividing fractions: 4 \div \frac16 = 4 \times \frac61 = 4\times 6 and we can see that there are 4 wholes with 6 sixths in each whole, so there are 4\times 6 = 24 sixths in 4.
-
How many two-thirds are in 2?

Each whole yields a two-thirds and one half of another two-thirds, therefore 3 sets of two-thirds can be made.
Write this as a division problem: 2 \div \frac23 = 3
We can connect this to the algorithm for dividing fractions: 2 \div \frac23 = 2 \times \frac32 = \frac{2 \times 3}{2} and we can see that there are 2 wholes with 3 thirds in each whole, so there are 2\times 3 thirds in 2. Because we want to know how many two-thirds there are, we have to make groups of 2 thirds, or divide the number of thirds we have by 2. So there are (2\times 3)\div 2 = 6\div 2 = 3 two-thirds in 2.
-
How many three-fourths are in 2?

2 complete sets of three-fourths can be made and 2 of the 3 pieces need to make \frac34 are left over, so we have another \frac23 of a three-fourths.
Write this as a division problem: 2 \div \frac34 = 2 \frac23
We can connect this to the algorithm for dividing fractions: 2 \div \frac34 = 2 \times \frac43 = \frac{2 \times 4}{3} and we can see that there are 2 wholes with 4 fourths in each whole, so there are 2\times 4 fourths in 2. Because we want to know how many three-fourths there are, we have to make groups of 3 fourths, or divide the number of fourths we have by 3. So there are (2\times 4)\div 3 = 8\div 3 = 2 \frac23 three-fourths in 2.
-
How many \frac16’s are in \frac13?

It takes two one-sixths to make a third.
Write this as a division problem. \frac13 \div \frac16 = 2
We can connect this to the algorithm for dividing fractions: \frac13 \div \frac16 = \frac13 \times \frac61 and we can see that there are 1 \times 6 = 6 sixths in a whole. The picture shows \frac13 of those 6 pieces. There are \frac13 \times 6 = 2 sixths in one-third.
-
How many \frac16’s are in \frac23?

It takes 4 one-sixth sized pieces to make the two-thirds.
Write this as a division problem:\frac23 \div \frac16 = 4
We can connect this to the algorithm for dividing fractions: \frac23 \div \frac16 = \frac23 \times \frac61 and we can see that there 1 \times 6 = 6 sixths in a whole. The picture shows \frac23 of those 6 pieces. There are \frac23 \times 6 = 4 sixths in two-thirds.
-
How many \frac14’s are in \frac23?

If the whole is divided into twelfths, four of them represent \frac13 and three of them represent \frac14 as shown in the picture above.

In left picture, we have \frac23, and in the right we have highlighted 2 twelfths that we are going to move to make it easier two see how many \frac14 there are in \frac23.
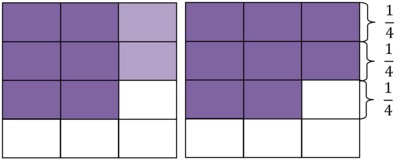
On the left we can see where those 2 twelfths were moved to, and on the right we have colored them normally so we can focus on how many fourths there are. There are 2 fourths, and \frac23 of another fourth. So all together, there are 2\frac23 fourths in \frac23.
Write this as a division problem: \frac23 \div \frac14 = 2 \frac23
We can connect this to the algorithm for dividing fractions: \frac23 \div \frac14 = \frac23 \times \frac41 = \frac{2\times4}{3\times1} and we can see that there 2 \times 4 = 8 twelfths in two-thirds. To find how many fourths there are, we need to see how many groups of 3 twelfths there are; in other words, there are 8\div 3 = 2\frac23 fourths in two-thirds.
-
How many \frac{5}{12}’s are in \frac12?

One complete set of five-twelfths will fit into a one-half but there is still a one-twelfth left over which is one of the five needed to make another set of five-twelfths.
Write this as a division problem: \frac12 \div \frac{5}{12} = 1 \frac15
We can connect this to the algorithm for dividing fractions, although with this picture it is a bit tricky: \frac12 \div \frac{5}{12} = \frac12 \times \frac{12}{5} =(\frac12 \times 12)\div 5 = \frac65 = 1\frac15 We can see in the picture that the whole is divided into 12 equal pieces and we are interested in dividing half of those pieces by 5.
In other words, (\frac12 of a whole \times 12 pieces in the whole) \div 5 of the twelve pieces in the set.
How many _______ are in. . . ?
Solve each problem using pictures and using a number sentence involving division.
- How many fives are in 15?
- How many halves are in 3?
- How many sixths are in 4?
- How many two-thirds are in 2?
- How many three-fourths are in 2?
- How many \frac16’s are in \frac13?
- How many \frac16’s are in \frac23?
- How many \frac14’s are in \frac23?
- How many \frac{5}{12}’s are in \frac12?
