Summer Intern
Task
You have been hired for a summer internship at a marine life aquarium. Part of your job is diluting brine for the saltwater fish tanks. The brine is composed of water and sea salt, and the salt concentration is 15.8% by mass, meaning that in any amount of brine the mass of salt is 15.8% of the total mass.
- The supervisor asks you to add fresh water to one liter of the brine using a half-liter measuring cup. Let S(x) be the salt concentration of the resulting mixture when you add x half-liters of salt. Write an expression for S(x). [Assume that one liter of water has mass 1 kg.]
- Describe how the graph of S is related to the graph of y = 1/x.
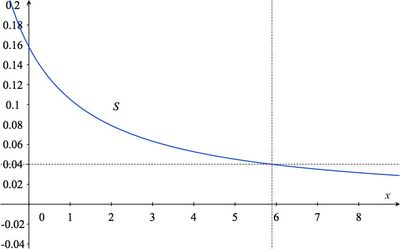
- Sketch the graph of S.
- How much fresh water should you add to get a mixture which is 4% sea salt, approximately the salt concentration of the ocean?
Solutions
Solution: Summer Intern
- Because the brine is 15.8% sea salt, the initial salt concentration is \frac{\mbox{0.158 kilograms of salt}}{\mbox{1 kilogram of brine}}.Adding x half-liters of water is the same as adding x half-kilograms of mass. This increases the total mass of the mixture by the x half-kilograms, to 0.5x+1, while leaving the mass of salt unchanged. So an expression for S(x) is \frac{0.158}{0.5x+1}
- We write the expression for S(x) in a form that shows its relation with 1/x:
\frac{0.158}{0.5x+1} = \frac{0.158}{0.5(x+2)} = \frac{0.316}{ x+2} = 0.316 \frac{1}{x+2}.Thus S(x) is obtained from 1/x by first replacing x with x+2 and then multiplying the whole by 0.316. So the graph of S is the graph of y={1\over x}horizontally translated by 2 units to the left, and vertically dilated by 0.316 units.
-

- Since a solution which is 4% sea salt has a salt concentration of 0.04, we must find x satisfying S(x) = 0.04. So, we solve the equation
\displaystyle{0.316\over x+2}=0.04 for x:
\begin{eqnarray*}
{0.316\over x+2}&=&0.04\\
0.316&=&0.04 (x+2)\\
\frac{0.316}{0.04}&=&x+2\\
7.9&=&x+2\\
5.9&=&x.
\end{eqnarray*}So to each liter of 15.8% brine we would have to add about 6 half-liters of fresh water (3 liters) in order to get a 4% sea salt solution.
Solution: A tabular approach to part (d)
Using a calculator or spreadsheet we construct a table of values of S(x) from the expression for S(x) found in (b):
| Number of half-liters | Salt concentration |
| 0 | 0.158 |
| 1 | 0.153 |
| 2 | 0.079 |
| 3 | 0.0632 |
| 4 | 0.0527 |
| 5 | 0.045 |
| 6 | 0.0395 |
| 7 | 0.035 |
The table shows that the salt concentration is very close to 4% when 6 half-liters (3 liters) of fresh water are added to the brine.
Solution: A graphical approach to part (d)
The solution to part (d) can be estimated from the graph found in (c) by drawing a horizontal line with vertical coordinate 0.04 and seeing what the value of x is where it intersects the graph of S:
Summer Intern
You have been hired for a summer internship at a marine life aquarium. Part of your job is diluting brine for the saltwater fish tanks. The brine is composed of water and sea salt, and the salt concentration is 15.8% by mass, meaning that in any amount of brine the mass of salt is 15.8% of the total mass.
- The supervisor asks you to add fresh water to one liter of the brine using a half-liter measuring cup. Let S(x) be the salt concentration of the resulting mixture when you add x half-liters of salt. Write an expression for S(x). [Assume that one liter of water has mass 1 kg.]
- Describe how the graph of S is related to the graph of y = 1/x.
- Sketch the graph of S.
- How much fresh water should you add to get a mixture which is 4% sea salt, approximately the salt concentration of the ocean?
