Zeroes and factorization of a non polynomial function
Task
- Sketch graphs of the functions f and F given by f(x)= |x| and F(x) = x^2 for -2 \leq x \leq 2.
- Suppose g is the function given by g(x) = \frac{f(x)}{x} for x \neq 0 and G is the function given by G(x) = \frac{F(x)}{x} for x \neq 0. Sketch graphs of the functions g and G for x \neq 0 and -2 \leq x \leq 2.
- Is there a natural way to define g and G when x =0? Explain.
IM Commentary
For a polynomial function f, if f(0) = 0 then the polynomial f(x) is divisible by x. This fact is shown and then generalized in ''Zeroes of a quadratic polynomial I, II'' and ''Zeroes of a general polynomial.'' Here, divisibility tells us that the quotient \frac{f(x)}{x} will still be a nice function -- indeed, another polynomial, save for the missing point at x=0. The goal of this task is to show via a concrete example that this nice property of polynomials is not shared by all functions. The non-polynomial function F given by F(x)=|x| is a familiar function for which property does not hold: even though F(0)=0, the quotient \frac{F(x)}{x} behaves badly near x=0. Indeed, its graph is broken into two parts which do not connect at x = 0.
The level of the task is appropriate for assessment but since its intention is to provide extra depth to the standard A-APR.2 it is principally designed for instructional purposes only. The students may use graphing technology: the focus, however, should be on what happens to the function g when x = 0 and the calculator may or may not be of help here (depending on how sophisticated it is!).
Solution
-
Below is a picture of the graph of the equation y = |x| when -2 \leq x \leq 2: it is the graph of the equation y = x when 0 \leq x \leq 2 and the graph of the equation y = -x when -2 \leq x \leq 0.

Below is a picture of the graph of the equation y = x^2 when -2 \leq x \leq 2:

-
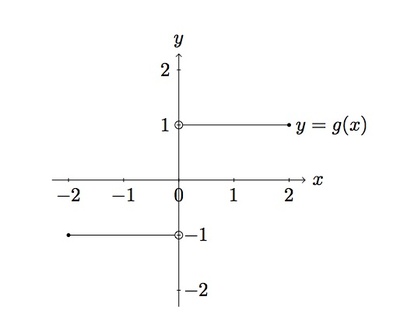
If g satisfies g(x) = \frac{f(x)}{x} this means that g(x) = \frac{|x|}{x}. When x \gt 0 we have |x| = x and so in this case g(x) = \frac{x}{x} = 1. Similarly, if x \lt 0 then |x| = -x and so g(x) = \frac{-x}{x} = -1. Below is a graph of the function y = g(x):
If G is a function which satisfies G(x) = \frac{F(x)}{x} this means that G(x)= \frac{x^2}{x} = x. The graph of G is shown below for -2 \leq x \leq 2 and x \neq 0:

-
The graph of g is broken into two parts which do not come together when x = 0. To the right of x = 0, g always takes the value 1 while to the left of x = 0, g always takes the value -1. Coming from the left g appears as if it will take the value -1 when x = 0 while coming from the right it appears as if g will take the value 1 when x = 0. There is no ''natural'' choice for what value g should take when x=0.
Unlike g(x), the function G(x) looks exactly like the function h(x) = x except that the point (0,0) is missing. This makes sense because G(x) = \frac{x^2}{x} = x and the only problem with G is that it has been expressed with an x in the denominator so that it is not defined when x = 0. Looking at the graph of G, however, it is natural to define G(0) = 0.
Zeroes and factorization of a non polynomial function
- Sketch graphs of the functions f and F given by f(x)= |x| and F(x) = x^2 for -2 \leq x \leq 2.
- Suppose g is the function given by g(x) = \frac{f(x)}{x} for x \neq 0 and G is the function given by G(x) = \frac{F(x)}{x} for x \neq 0. Sketch graphs of the functions g and G for x \neq 0 and -2 \leq x \leq 2.
- Is there a natural way to define g and G when x =0? Explain.
