Constant Speed

Task

Callie biked 12 miles in 3 hours. Carter biked 10 miles in 2 hours.

Represent each person's trip with a diagram. Explain how you can see that they are not going the same speed.

IM Commentary

The purpose of this task is for students to learn to reason about whether or not ratios are equivalent using a diagram. If students are familiar with different types of diagrams, they might be left to decide on an appropriate representation (MP.5). Or a teacher might use this task to practice or promote the use of a particular type of diagram. The type of diagram shown in the solution is a parallel tape diagram, but a double number line diagram or a ratio table could also work nicely if students are familiar with them.

The brief nature of the task statement might suggest this is a nice, short problem for students, but the reasoning could take some time.

If a teacher anticipates that students might struggle to get started, she might preface the task statement with an example using a preferred representation. So before students tackle this task, the class might work to make sense of a statement like, "If you walked 9 miles in 3 hours, we might represent that situation with this diagram."

The teacher could ask students what they notice, or ask more specific questions like,

• How much time did it take you to walk 6 miles? [2 hours]
• About how far had you walked after an hour and a half? [$4\frac{1}{2}$ miles]
• If someone were walking slower than you, what would have to be true? [Answers will vary, but involve walking a shorter distance in the same time (8 miles in 3 hours), or taking more time to walk the same distance (9 miles in 4 hours).]

For students who might finish early, some appropriate extension questions might be:

• Suppose someone biked $15\frac{1}{2}$ miles in 4 hours. Is this person going faster or slower than the people in the task? Explain how you know. [This person's speed is $3\frac{7}{8}$ miles per hour, so it's slower than both people in the task.]
• Write a different story about two people biking different distances at the same speed, and draw a diagram that shows how you know they are traveling the same speed.
• Draw a diagram that shows a person biking at a certain speed for one hour, then at a faster speed for the next hour, and then at an even faster speed for the third hour.

The task makes an assumption that each cyclist is traveling at a constant speed. If it comes up, a teacher could just say, "Let's assume that they each travel the same speed for the whole ride."

Solution

Here is a diagram representing Callie's ride:

Here is a diagram representing Carter's ride:

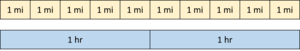
Sample reasoning based on comparing the same amount of time: In the first diagram, you can see Callie rides 4 miles in one hour. In the second diagram you can see that Carter rides 5 miles in one hour. Since they each travel a different distance in the same amount of time, they are not traveling at the same speed.

Sample reasoning based on comparing the same distance: In the first diagram, you can see that Callie takes 1 hour to travel 4 miles. But in the second diagram, you can see that Carter takes less than one hour to travel 4 miles. Since it takes them a different amount of time to travel the same distance, they are not traveling at the same speed.