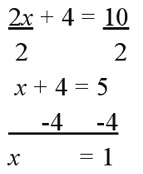Solving Equations
In elementary school, students often draw pictures of the arithmetic they do. For instance, they might draw the following picture for the problem 2 + 3:
In this picture, each square represents a tile.
We can do the same thing for algebraic expressions, but we need to be careful about how we represent the unknown. If we assume that an unknown number of tiles x are contained in a bag, we could draw the following picture for 2x + 3:

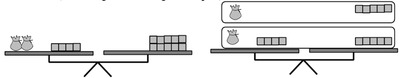
When we have an equation to solve, we assume that the two sides of the equation are equal. We can represent this by showing them level on a balance. For example, we the equation 2x + 3 = 7 could be shown as:
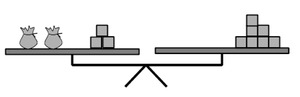
When we solve equations, we can add, subtract, multiply or divide both sides of the equation by the same thing in order to maintain the equality. This can be shown in pictures by keeping the balance level. For example, we could solve the equation 2x + 3 = 7 using pictures by first removing (subtracting) 3 from each side, and then splitting (dividing) the remaining blocks into two equal groups:
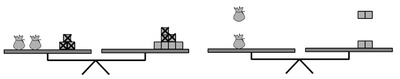
From this picture, we can see that, in order to keep the balance level, each bag must contain 2 tiles, which means that x = 2.
-
Solve 5x + 1 = 2x + 7 in two ways: symbolically, the way you usually do with equations, and also with pictures of a balance. Show how each step you take symbolically is shown in the pictures.
-
Solve the equation 4x = x + 1 using pictures and symbols. Discuss any issues that arise.
-
What issues arise when you try to solve the equation 2 = 2x - 4 using pictures? Do the same issues arise when you solve this equation symbolically?
-
Make up a linear equation that has no solutions. What would happen if you solved this equation with pictures? How is this different than an equation that has infinitely many solutions?
Use pictures to show why the following solution to the equation 2x + 4 = 10 is incorrect: