Introduction to Linear Functions

Task

1. Decide which of the following points are on the graph of the function $y = 2x + 1$:

   • $(0, 1), (2, 5), (\frac12, 2), (2, −1), (−1, −1), (0.5, 1)$.
   • Find 3 more points on the graph of the function.

2. Find several points that are on the graph of the function $y = 2x^2 + 1$.

   • Plot the points in the coordinate plane. Is this a linear function?
   • Support your conclusion.
3. Graph both functions and list as many differences between the two functions as you can.

IM Commentary

This task lets students explore the differences between linear and non-linear functions. By contrasting the two, it reinforces properties of linear functions.

The task lends itself to an extended discussion comparing the differences that students have found and relating them back to the equation and the graph of the two functions. The teacher can use the opportunity to draw triangles representing the horizontal and vertical change between points on the graph to illustrate the constant slope of the line vs. the varying rate of change of the quadratic function.

Some of the differences in the list of part (c) generalize to all linear and quadratic functions, other do not. It would be worth discussing which are properties of the type of function and which are not.

Solution

1. We can find which of the given points are on the line $y = 2x + 1$ by seeing if the $x$ and $y$ coordinate satisfy the equation. For the first point, with $y = 1$ and $x = 0$, we see

   $$ 1 = 2(0) + 1 = 1 $$

   which is true, and so the point $(0,1)$ is on the line. However, for $(2,-1)$, by substituting $x = 2$ and $y = -1$, we have

   $$ -1 \neq 2(2) + 1 = 5 $$

   and so $(2,-1)$ is not on the line.

   Continuing this way, we see that $(0,1), (2,5), (1/2,2)$, and $(-1,-1)$ are points on the line and $(2,-1)$, and $(.5,1)$ are not points on the line.

   We can find three more points by arbitrarily choosing value for $x$ and using the equation for the line to find the corresponding $y$ values: $(-2, -3), (1, 3), (-1/2, 0)$.

2. By picking arbitrary $x$ values, such as $x=0$, $x=1$, $x=2$, $x=-1$, and $x=-2$, we find the corresponding $y$ values and have several points that lie on the graph of the function:

   $$ (0, 1), (1, 3), (2, 9), (-1, 3), (-2, 9) $$

   By plotting these points, we arrive at the following graph:

   

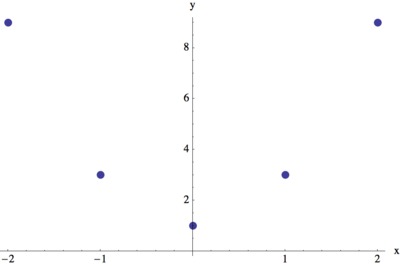
   A linear function can be written in the form $y=mx+b$, and this is equation is not written in that form. We might wonder whether it could be written in that form using some clever trick we haven't thought of yet. If it were a linear function, its graph would be a straight line. The graph of $y = 2x^2 + 1$ contains the above five points, and these five points do not lie in a line. Thus, it is not a linear function.

   

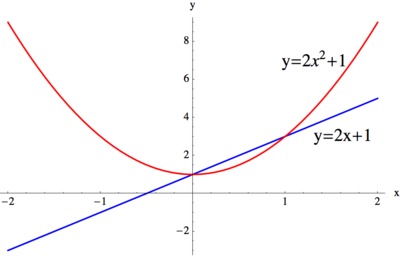
3. Below is a list of differences between these two functions. Possible answers may include some, all, or more differences.

   • The first graph is a straight line, the second graph is curved.
   • The $x$ term is squared in the second function, and not in the first.
   • The first function has negative and positive $y$ values, and the second function will never have negative $y$ values.
   • As $x$ increases by equal amounts, the $y$ values in the first function also increase by equal amounts (rate of change is constant) but the $y$ values of the second function increase by bigger and bigger amounts (rate of change is increasing) as $|x|$ gets larger.
   • The first function has a constant slope (steepness). The steepness of the second function changes.
   • The second function is symmetrical about the $y$-axis, and the first function is not.
   • The first function crosses the $x$-axis (at $(-1/2,0)$), and the second function never crosses the $x$-axis.
   • Each $y$ value in the first function has exactly one $x$ value. In the second function, most $y$ values have 2 possible $x$ values (example: $(-2,9)$ and $(2,9)$ both lie on the second graph).