Fixed points of rigid motions

Task

A rigid motion of the plane is a map of the plane to itself which preserves distances between points. Let $f$ be such a function.A point $x$ in the plane is called a fixed point of the rigid motion $f$ if $f(x) = x$.

1. Suppose $f$ is the map which translates $A$ to $B$ where $A$ and $B$ are distinct points in the plane. What are the fixed points of $f$? Explain.

2. Suppose $g$ is the map which rotates the plane by 45 degrees counterclockwise around a point $P$. What are the fixed points of $g$? Explain.
3. Suppose $h$ is the map which reflects the plane about a line $\ell$. What are the fixed points of $h$?

4. Suppose $t$ is any rigid motion of the plane. Explain why there are four possibilities for the set of fixed points of $t$:

   • no points
   • a single point
   • a line
   • all points.
   For each of the four possibiilties, give an example of a transformation whose fixed points match the description.

IM Commentary

The purpose of this task is to use fixed points at a tool for studying and classifying rigid motions of the plane. In particular, the three basic types of rigid motions (translations, rotations, and reflections) are characterized by the collection of points that they fix. If the students are used to and comfortable working with Cartesian coordinates in the plane, the teacher may wish to supplement this task by working with ${\bf R}^2$ in addition to $E$. For this problem, however, the exact coordinates are not used and finding the functions for (b) and (c) can be very challenging in a coordinate system: thus this task provides an opportunity to see a situation (translations) where the coordinate approach works very naturally and also two situations (reflections and rotations) where the coordinate approach can be quite difficult. It is therefore important for students to be able to work both with and without coordinates.

Ideally students are already familiar with the mathematical definitions of translations, rotations, and reflections. In the solution below, these definitions are used for rotations and reflections. Intuitive arguments with pictures can also be used and sample pictures are also provided in the solution. If the teacher desires a more open ended version of this task, part (d) can be used on its own.

A thorough study of fixed points can help provide a classification of rigid motions of the plane. The only possibilities for the set of fixed points of a rigid motion are, as shown in part (d), all of $E$, a line $\ell$, a single point $P$, or no points. With some more work it is possible to classify these cases as follows:

• If all points are fixed, then the rigid motion is the identity.
• If there is a line $\ell$ of fixed points, then the rigid motion is reflection about $\ell$.
• If there is a single fixed point $P$, then the rigid motion is a rotation about $P$ by an angle which is not an integer multiple of 360 degrees.
• If there are no fixed points, then the rigid motion is either a translation (which is not the identity) or a ''glide reflection'' which is a translation (which is not the identity) followed by a reflection about a line parallel to the direction of translation.

This line of reasoning extends part (d) of the task. Although it goes beyond the high school curriculum, this could make for a nice project in an advanced geometry class.

Solution

1. The translation map $f$ which sends $A$ to $B$ moves all points by the same distance and in the same direction. So for any point $C \in E$ we have $$ |Cf(C)| = |AB| \neq 0. $$ This means that $f(C) \neq C$ and so $f$ has no fixed points. A sample is pictured below for the case where $C$ does not lie on $\overleftrightarrow{AB}$:

   

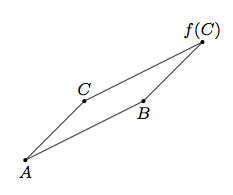
2. The definition of rotation about $P$ tells us that the center of the rotation does not move, that is $g(P) = P$. Thus $P$ is a fixed point of $g$. If $Q \neq P$ is some other point in $E$ then $Q$ maps to $R = f(Q)$ where $|PR| = |QR|$ and $m(\angle QPR) = 45$. This is pictured below and we have that $R = f(Q) \neq Q$ so $P$ is the only fixed point of $g$.

   

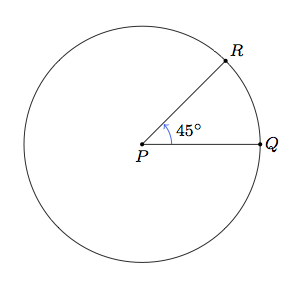
3. The definition of reflection about $\ell$ tells us that $h(Q) = Q$ if $Q \in \ell$ so every point on $\ell$ is fixed. If $Q \notin \ell$ then $h(Q) = R$ where $\ell$ is the perpendicular bisector of $\overline{QR}$. In particular, $Q$ and $h(Q)$ are on opposite sides of $\ell$, as pictured below, and so they are distinct. This means that the only fixed points of $h$ are the points on $\ell$.

   

4. Suppose $t$ is any transformation of the plane. We have already seen in part (a) that a translation has no fixed points and in part (b) that a rotation (by an angle which is not a multiple of 360$^\circ$) has a single fixed point. We also saw in part (c) that a reflection fixes all points on a line. Finally, the identity transformation maps every point to itself and so all points are fixed by the identity. This shows that the four possibilities listed (no points, one point, a line, all points) all occur.

   Now we must argue that these are the only possibilities. Suppose, then that $t$ fixes two distinct points $A$ and $B$. This means that $t(A) = A$ and $t(B) = B$. If $C$ is a point in the plane then $|t(A)t(C)| = |AC|$ and $|t(B)t(C)| = |BC|$ since $t$ preserves distances. This means that

   $$ \begin{align} |At(C)| &= |AC| \\ |Bt(C)| &= |BC| \end{align} $$

   If $C$ lies on $\overleftrightarrow{AB}$ then its position is determined by its distance from $A$ and its distance from $B$. So the two equations above tell us that $t(C) = C$.

   We have shown that if $t$ fixes two distinct points $A$ and $B$, it must fix all points on $\overleftrightarrow {AB}$. If, in addition, $t$ fixes a third point $C$ not collinear with $A$ and $B$, then it also fixes all points on $\overleftrightarrow{AC}$ and $\overleftrightarrow{BC}$. Applying this argument again, $t$ fixes all points on all lines connecting a pair of points chosen from $\overleftrightarrow{AB}$, $\overleftrightarrow{AC}$, or $\overleftrightarrow{BC}$. But these lines cover the entire plane and so $t$ is the identity, fixing all points.

   Our argument shows that as soon as $t$ fixes two distinct points, it fixes the line joining them and as soon as it fixes three non collinear points, it fixes all points. So the only possible fixed point sets for $t$ are the four described: no points, one point, a line, or all points.