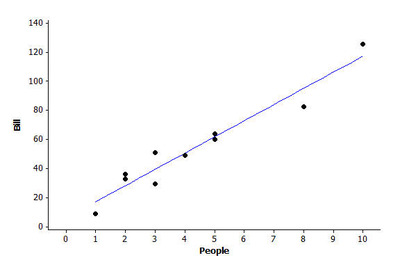
Restaurant Bill and Party Size

Task

The owner of a local restaurant selected a random sample of dinner tables at his restaurant. For each table, the owner recorded the total amount of the dinner bill and the number of people at the table. The data are given in the table below. A scatterplot of the data is also shown.

People	Bill
1	$8.50
2	$36.00
2	$32.55
3	$29.30
3	$50.65
4	$48.75
5	$63.75
5	$60.00
8	$82.50
10	$125.75

 

1. Does the relationship between number of people and the bill appear to be weak, moderate or strong?

2. Does the relationship between number of people and the bill appear to be linear?

3. The equation of the line of best fit is Bill = 5.80 + 11.15 · People. Sketch this line on the scatterplot.

4. Interpret the slope of the line of best fit in the context of this problem.

5. Note that the points in the scatterplot do not all lie on the line. What could explain this variability?

6. Use the equation of the line of best fit to estimate how much would you predict the bill to be for a party of 8?

7. There was one party of 8 whose bill was $82.50. Did they pay more than or less than the predicted amount? How much more or how much less did they pay?

8. The difference calculated in part (g) is called a residual. When each residual is calculated and plotted against the corresponding number of people, the resulting plot is called a residual plot. The residual plot for this data set is shown below.

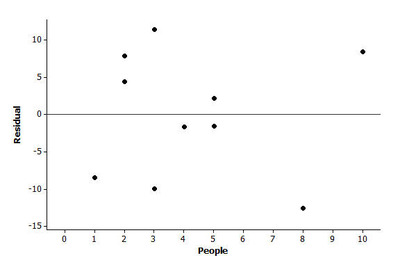
Explain how one could use the residual plot in order to determine if a linear model is an appropriate way to describe the relationship between number of people and the amount of the bill.

i.  A random sample of several pizza restaurants in the area was selected. Data was collected on the diameter and the price of the smallest cheese pizza sold at the restaurant. The line of best fit was fit to the data and the corresponding residual plot is given below.     

 Does there appear to be a linear relationship between the diameter and the price of a cheese pizza in this area? Use the residual plot to explain your answer.

 

IM Commentary

The purpose of this task is to assess student understanding of residuals and residual plots (S.ID.6). Students see a residual plot in a context where a linear model is appropriate and also see a residual plot in a context where a linear model is not appropriate. 

Points to include in the discussion of this task are

• Positive residuals correspond to points that lie above the linear model and negative residuals lie below the linear model.

• If a linear model is appropriate, we would expect the residuals to represent random deviations from a line and so we would not expect to see any systematic pattern in the residual plot.

• The residual plot in part (i) shows a distinct pattern and indicates that it is not appropriate to use a linear model to describe the relationship between diameter and price.

 

Solution

a, b.  This relationship appears to be fairly strong and linear. The points do not stray far from a line.

    c.

    d.  Each additional person added to the party adds approximately $11.15 to the bill.

e.  This variability can be explained by differences in what the parties ordered to eat or drink. For example, we would not expect one party of 5 people to order food that costs exactly the same as every other party of 5 people.

     f.  5.80 + 11.15(8) = 95 dollars  

    g.  This party of 8 paid $12.50 ($95 - $82.50 = $12.50) less than the predicted amount.

    h.  If a linear model is appropriate, we would expect to see a random scattering of points about the horizontal axis. That is the case for the residual plot shown here—there is no obvious pattern in the residual plot.

     i.  Because of the “U shape” we see in the residual plot, we would expect the scatterplot to be concave upward.